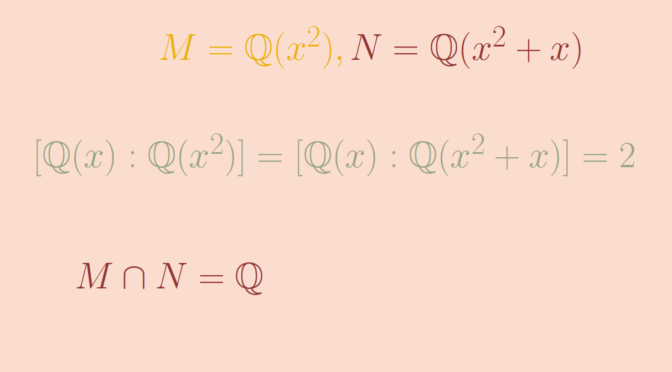Let’s describe an example of a field \(K\) which is of degree \(2\) over two distinct subfields \(M\) and \(N\) respectively, but not algebraic over \(M \cap N\).
Let \(K=F(x)\) be the rational function field over a field \(F\) of characteristic \(0\), \(M=F(x^2)\) and \(N=F(x^2+x)\). I claim that those fields provide the example we’re looking for.
\(K\) is of degree \(2\) over \(M\) and \(N\)
The polynomial \(\mu_M(t)=t^2-x^2\) belongs to \(M[t]\) and \(x \in K\) is a root of \(\mu_M\). Also, \(\mu_M\) is irreducible over \(M=F(x^2)\). If that wasn’t the case, \(\mu_M\) would have a root in \(F(x^2)\) and there would exist two polynomials \(p,q \in F[t]\) such that \[
p^2(x^2) = x^2 q^2(x^2)\] which cannot be, as can be seen considering the degrees of the polynomials of left and right hand sides. This proves that \([K:M]=2\). Considering the polynomial \(\mu_N(t)=t^2-t-(x^2+x)\), one can prove that we also have \([K:N]=2\).
We have \(M \cap N=F\)
The mapping \(\sigma_M : x \mapsto -x\) extends uniquely to an \(F\)-automorphism of \(K\) and the elements of \(M\) are fixed under \(\sigma_M\). Similarly, the mapping \(\sigma_N : x \mapsto -x-1\) extends uniquely to an \(F\)-automorphism of \(K\) and the elements of \(N\) are fixed under \(\sigma_N\). Also \[
(\sigma_N\circ\sigma_M)(x)=\sigma_N(\sigma_M(x))=\sigma_N(-x)=-(-x-1)=x+1.\] An element \(z=p(x)/q(x) \in M \cap N\) where \(p(x),q(x)\) are coprime polynomials of \(K=F(x)\) is fixed under \(\sigma_M \circ \sigma_N\). Therefore following equality holds \[
\frac{p(x)}{q(x)}=z=(\sigma_2\circ\sigma_1)(z)=\frac{p(x+1)}{q(x+1)},\] which is equivalent to \[
p(x)q(x+1)=p(x+1)q(x).\] By induction, we get for \(n \in \mathbb Z\) \[
p(x)q(x+n)=p(x+n)q(x).\] Assume \(p(x)\) is not a constant polynomial. Then it has a root \(\alpha\) in some finite extension \(E\) of \(F\). As \(p(x),q(x)\) are coprime polynomials, \(q(\alpha) \neq 0\). Consequently \(p(\alpha+n)=0\) for all \(n \in \mathbb Z\) and the elements \(\alpha +n\) are all distinct as the characteristic of \(F\) is supposed to be non zero. This implies that \(p(x)\) is the zero polynomial, in contradiction with our assumption. Therefore \(p(x)\) is a constant polynomial and \(q(x)\) also according to a similar proof. Hence \(z\) is constant as was supposed to be proven.
Finally, \(K=F(x)\) is not algebraic over \(F=M \cap N\) as \((1,x, x^2, \dots, x^n, \dots)\) is independent over the field \(F\) which concludes our claims on \(K, M\) and \(N\).