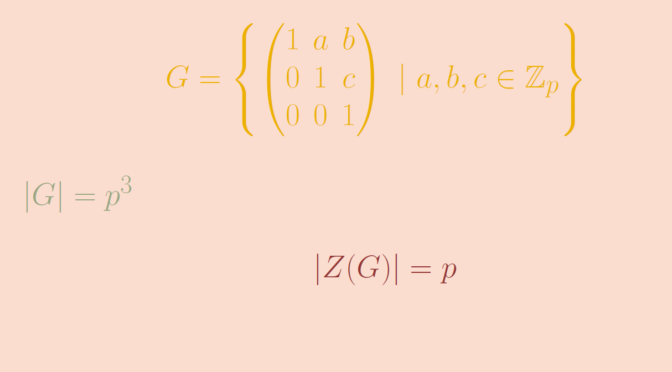Monthly Archives: February 2017

Algebra

A nonabelian \(p\)-group

Leave a comment

Consider a prime number \(p\) and a finite p-group \(G\), i.e. a group of order \(p^n\) with \(n \ge 1\).

If \(n=1\) the group \(G\) is cyclic hence abelian.

For \(n=2\), \(G\) is also abelian. This is a consequence of the fact that the center \(Z(G)\) of a \(p\)-group is non-trivial. Indeed if \(\vert Z(G) \vert =p^2\) then \(G=Z(G)\) is abelian. We can’t have \(\vert Z(G) \vert =p\). If that would be the case, the order of \(H=G / Z(G) \) would be equal to \(p\) and \(H\) would be cyclic, generated by an element \(h\). For any two elements \(g_1,g_2 \in G\), we would be able to write \(g_1=h^{n_1} z_1\) and \(g_2=h^{n_1} z_1\) with \(z_1,z_2 \in Z(G)\). Hence \[
g_1 g_2 = h^{n_1} z_1 h^{n_2} z_2=h^{n_1 + n_2} z_1 z_2= h^{n_2} z_2 h^{n_1} z_1=g_2 g_1,\] proving that \(g_1,g_2\) commutes in contradiction with \(\vert Z(G) \vert < \vert G \vert\). However, all \(p\)-groups are not abelian. For example the unitriangular matrix group \[
U(3,\mathbb Z_p) = \left\{
\begin{pmatrix}
1 & a & b\\
0 & 1 & c\\
0 & 0 & 1\end{pmatrix} \ | \ a,b ,c \in \mathbb Z_p \right\}\] is a \(p\)-group of order \(p^3\). Its center \(Z(U(3,\mathbb Z_p))\) is \[
Z(U(3,\mathbb Z_p)) = \left\{
\begin{pmatrix}
1 & 0 & b\\
0 & 1 & 0\\
0 & 0 & 1\end{pmatrix} \ | \ b \in \mathbb Z_p \right\},\] which is of order \(p\). Therefore \(U(3,\mathbb Z_p)\) is not abelian.

Analysis

Raabe-Duhamel’s test

Leave a comment

The Raabe-Duhamel’s test (also named Raabe’s test) is a test for the convergence of a series \[
\sum_{n=1}^\infty a_n \] where each term is a real or complex number. The Raabe-Duhamel’s test was developed by Swiss mathematician Joseph Ludwig Raabe.

It states that if:

\[\displaystyle \lim _{n\to \infty }\left\vert{\frac {a_{n}}{a_{n+1}}}\right\vert=1 \text{ and } \lim _{{n\to \infty }} n \left(\left\vert{\frac {a_{n}}{a_{{n+1}}}}\right\vert-1 \right)=R,\]
then the series will be absolutely convergent if \(R > 1\) and divergent if \(R < 1\). First one can notice that Raabe-Duhamel's test maybe conclusive in cases where ratio test isn't. For instance, consider a real \(\alpha\) and the series \(u_n=\frac{1}{n^\alpha}\). We have \[ \lim _{n\to \infty } \frac{u_{n+1}}{u_n} = \lim _{n\to \infty } \left(\frac{n}{n+1} \right)^\alpha = 1\] and therefore the ratio test is inconclusive. However \[ \frac{u_n}{u_{n+1}} = \left(\frac{n+1}{n} \right)^\alpha = 1 + \frac{\alpha}{n} + o \left(\frac{1}{n}\right)\] for \(n\) around \(\infty\) and \[ \lim _{{n\to \infty }} n \left(\frac {u_{n}}{u_{{n+1}}}-1 \right)=\alpha.\] Raabe-Duhamel's test allows to conclude that the series \(\sum u_n\) diverges for \(\alpha <1\) and converges for \(\alpha > 1\) as well known.

When \(R=1\) in the Raabe’s test, the series can be convergent or divergent. For example, the series above \(u_n=\frac{1}{n^\alpha}\) with \(\alpha=1\) is the harmonic series which is divergent.

On the other hand, the series \(v_n=\frac{1}{n \log^2 n}\) is convergent as can be proved using the integral test. Namely \[
0 \le \frac{1}{n \log^2 n} \le \int_{n-1}^n \frac{dt}{t \log^2 t} \text{ for } n \ge 3\] and \[
\int_2^\infty \frac{dt}{t \log^2 t} = \left[-\frac{1}{\log t} \right]_2^\infty = \frac{1}{\log 2}\] is convergent, while \[
\frac{v_n}{v_{n+1}} = 1 + \frac{1}{n} +\frac{2}{n \log n} + o \left(\frac{1}{n \log n}\right)\] for \(n\) around \(\infty\) and therefore \(R=1\) in the Raabe-Duhamel’s test.

Algebra

Subset of elements of finite order of a group

Leave a comment

Consider a group \(G\) and have a look at the question: is the subset \(S\) of elements of finite order a subgroup of \(G\)?

The answer is positive when any two elements of \(S\) commute. For the proof, consider \(x,y \in S\) of order \(m,n\) respectively. Then \[
\left(xy\right)^{mn} = x^{mn} y^{mn} = (x^m)^n (y^n)^m = e\] where \(e\) is the identity element. Hence \(xy\) is of finite order (less or equal to \(mn\)) and belong to \(S\).

Example of a non abelian group

In that cas, \(S\) might not be subgroup of \(G\). Let’s take for \(G\) the general linear group over \(\mathbb Q\) (the set of rational numbers) of \(2 \times 2\) invertible matrices named \(\text{GL}_2(\mathbb Q)\). The matrices \[
A = \begin{pmatrix}0&1\\1&0\end{pmatrix},\ B=\begin{pmatrix}0 & 2\\\frac{1}{2}& 0\end{pmatrix}\] are of order \(2\). They don’t commute as \[
AB = \begin{pmatrix}\frac{1}{2}&0\\0&2\end{pmatrix} \neq \begin{pmatrix}2&0\\0&\frac{1}{2}\end{pmatrix}=BA.\] Finally, \(AB\) is of infinite order and therefore doesn’t belong to \(S\) proving that \(S\) is not a subgroup of \(G\).

Analysis

Counterexamples around Cauchy condensation test

1 Comment

According to Cauchy condensation test: for a non-negative, non-increasing sequence \((u_n)_{n \in \mathbb N}\) of real numbers, the series \(\sum_{n \in \mathbb N} u_n\) converges if and only if the condensed series \(\sum_{n \in \mathbb N} 2^n u_{2^n}\) converges.

The test doesn’t hold for any non-negative sequence. Let’s have a look at counterexamples.

A sequence such that \(\sum_{n \in \mathbb N} u_n\) converges and \(\sum_{n \in \mathbb N} 2^n u_{2^n}\) diverges

Consider the sequence \[
u_n=\begin{cases}
\frac{1}{n} & \text{ for } n \in \{2^k \ ; \ k \in \mathbb N\}\\
0 & \text{ else} \end{cases}\] For \(n \in \mathbb N\) we have \[
0 \le \sum_{k = 1}^n u_k \le \sum_{k = 1}^{2^n} u_k = \sum_{k = 1}^{n} \frac{1}{2^k} < 1,\] therefore \(\sum_{n \in \mathbb N} u_n\) converges as its partial sums are positive and bounded above. However \[\sum_{k=1}^n 2^k u_{2^k} = \sum_{k=1}^n 1 = n,\] so \(\sum_{n \in \mathbb N} 2^n u_{2^n}\) diverges.

A sequence such that \(\sum_{n \in \mathbb N} v_n\) diverges and \(\sum_{n \in \mathbb N} 2^n v_{2^n}\) converges

Consider the sequence \[
v_n=\begin{cases}
0 & \text{ for } n \in \{2^k \ ; \ k \in \mathbb N\}\\
\frac{1}{n} & \text{ else} \end{cases}\] We have \[
\sum_{k = 1}^{2^n} v_k = \sum_{k = 1}^{2^n} \frac{1}{k} – \sum_{k = 1}^{n} \frac{1}{2^k} > \sum_{k = 1}^{2^n} \frac{1}{k} -1\] which proves that the series \(\sum_{n \in \mathbb N} v_n\) diverges as the harmonic series is divergent. However for \(n \in \mathbb N\), \(2^n v_{2^n} = 0 \) and \(\sum_{n \in \mathbb N} 2^n v_{2^n}\) converges.